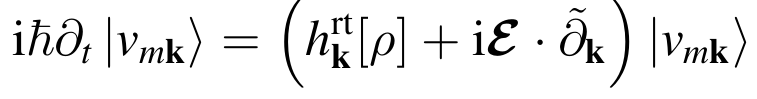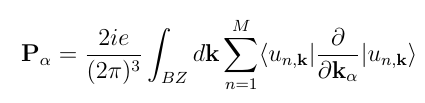Introduction to Real Time propagation in Yambo
Time Dependent Equation for the Reduced One--Body Density--Matrix
Time Dependent Effective Schrödinger Equation
The Yambo code implements a Time Dependent Effective Schrödinger Equation(TD-ESE), where the coupling between electrons and the external field is described by means of the Modern Theory of Polarization:
Where [math]\displaystyle{ | v_{i \mathbf{k}} \rangle }[/math] are the time-dependent valence bands, Hsysk is the Hamiltonian, [math]\displaystyle{ \mathcal E(t) }[/math] is the external field and the term [math]\displaystyle{ i e \partial k }[/math] corresponds to the dipole operator in periodic systems. For more details on this last term see Ref. [1] and [2].
Differently from other codes, the equations of motion of Yambo are in the length gauge and not in the velocity one. If you want to know more about the advantages and disadvantages of the two gauges read section 2.7 of Ref. [3].
The Hamiltonian and the initial wave-functions are obtained from DFT. Then in order to obtain linear response, we probe the system with a delta function field, that excites all the frequencies at the same footing, and Fourier transforms. We calculate the Berry polarization
and from its Fourier transform we get the linear response, see Ref. [2].
- ↑ PRB 69, 085106 (2004)
- ↑ 2.0 2.1 C. Attaccalite and M. Gruning Rev. B, 88, 235113 (2013)
- ↑ Non-linear response in extended systems: a real-time approach