Second-harmonic generation of 2D-hBN: Difference between revisions
No edit summary |
|||
| Line 1: | Line 1: | ||
= Step 0: Theoretical framework = | = Step 0: Theoretical framework = | ||
In this tutorial, we compute the Second-harmonic generation (SHG) from the dynamics of the Bloch-states. The equation-of-motion of the Bloch-states is explained in the tutorial on [[Linear response from Bloch-states dynamics]]. When we want to calculate the SHG, what changes with respect to that tutorial where we computed the dielectric function is: | |||
* the time-dependence of the input electric field and | |||
* the post-processing of the signal | |||
'''Time dependence of the electric Field''': we choose a sinusoidal electric field, thus a monochromatic laser field. This allows one to expand the polarization in the form <math>\bf{P}(t) = \sum_{n=-\infty}^{+\infty} \bf{p}_n e^{-i\omega_n t} </math> where the coefficient <math>\bf{p}_1,...,\bf{p}_n </math> are related to <math>\chi^{(1)},...,\chi^{(n)} </math> through a coefficient depending on a power of the strength of the field (with the power depending on the order of the response). | |||
At difference with a delta-like perturbation, a real-time simulation gives the response at the laser-field only. Then, to obtain the spectrum for the desired range of frequency, we have to perform so many simulations as the frequencies in the desired range. | |||
'''Post-processing of the signal''': The switch-on of the electric field corresponds to a weak delta-like kick. Thus, even if it may not be noticeable, the polarization results from both the sinusoidal field and the delta-like kick. The latter excites all eigenfrequencies of the system. Though weak, the resulting signal is comparable with the second-harmonic signal. To eliminate the signal from the eigenfrequencies, we apply a dephasing (<math>\gamma_{deph}</math>). To have a signal useful to sample the second-harmonic signal, we need to wait a time <math>\bar t</math> much larger than the dephasing-time <math>1/\gamma_{deph} </math>. Note that we cannot choose a too short dephasing time (to shorten the simulation time), as this would result in a too large broadening of the spectrum. After <math>\bar t</math>, we sample $2N+1$ times in a period and use discrete Fourier transform to extract <math>{p}_n</math> for <math> n = 0,...,N</math>, where n=2 gives the SHG. This is schematically illustrated in figure: | |||
[[File:Pt analysis.png|600px|center|Non-linear response analysis]] | |||
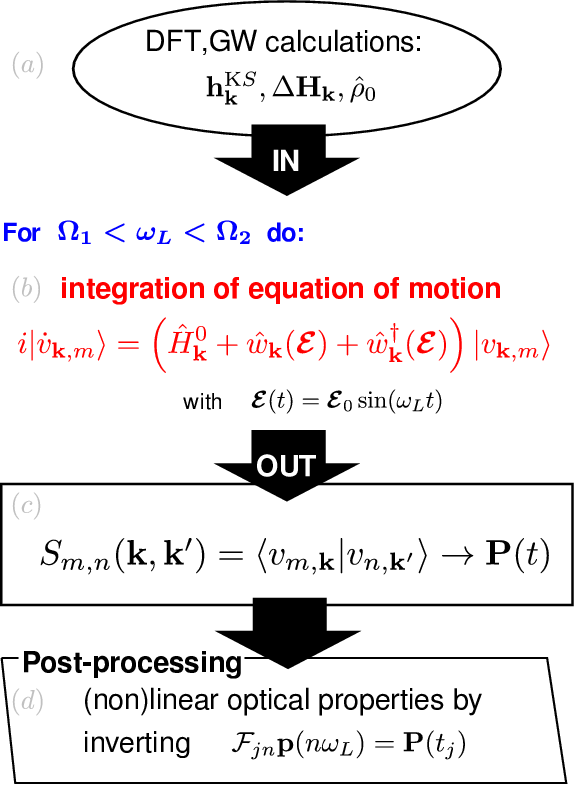
The scheme from Ref. <ref name="Attaccalite2013">C. Attaccalite and M. Gruning [https://arxiv.org/abs/1309.4012v2 Rev. B, '''88''', 235113 (2013)]</ref> summarised the workflow for computing the SHG (or higher-harmonics) in a energy range <math>[\Omega_1,\Omega_2]</math>. | |||
[[File:Scheme nl.png|350px|center|Schematic representation of real-time calculations]] | [[File:Scheme nl.png|350px|center|Schematic representation of real-time calculations]] | ||
Revision as of 14:24, 19 May 2023
Step 0: Theoretical framework
In this tutorial, we compute the Second-harmonic generation (SHG) from the dynamics of the Bloch-states. The equation-of-motion of the Bloch-states is explained in the tutorial on Linear response from Bloch-states dynamics. When we want to calculate the SHG, what changes with respect to that tutorial where we computed the dielectric function is:
- the time-dependence of the input electric field and
- the post-processing of the signal
Time dependence of the electric Field: we choose a sinusoidal electric field, thus a monochromatic laser field. This allows one to expand the polarization in the form [math]\displaystyle{ \bf{P}(t) = \sum_{n=-\infty}^{+\infty} \bf{p}_n e^{-i\omega_n t} }[/math] where the coefficient [math]\displaystyle{ \bf{p}_1,...,\bf{p}_n }[/math] are related to [math]\displaystyle{ \chi^{(1)},...,\chi^{(n)} }[/math] through a coefficient depending on a power of the strength of the field (with the power depending on the order of the response).
At difference with a delta-like perturbation, a real-time simulation gives the response at the laser-field only. Then, to obtain the spectrum for the desired range of frequency, we have to perform so many simulations as the frequencies in the desired range.
Post-processing of the signal: The switch-on of the electric field corresponds to a weak delta-like kick. Thus, even if it may not be noticeable, the polarization results from both the sinusoidal field and the delta-like kick. The latter excites all eigenfrequencies of the system. Though weak, the resulting signal is comparable with the second-harmonic signal. To eliminate the signal from the eigenfrequencies, we apply a dephasing ([math]\displaystyle{ \gamma_{deph} }[/math]). To have a signal useful to sample the second-harmonic signal, we need to wait a time [math]\displaystyle{ \bar t }[/math] much larger than the dephasing-time [math]\displaystyle{ 1/\gamma_{deph} }[/math]. Note that we cannot choose a too short dephasing time (to shorten the simulation time), as this would result in a too large broadening of the spectrum. After [math]\displaystyle{ \bar t }[/math], we sample $2N+1$ times in a period and use discrete Fourier transform to extract [math]\displaystyle{ {p}_n }[/math] for [math]\displaystyle{ n = 0,...,N }[/math], where n=2 gives the SHG. This is schematically illustrated in figure:
The scheme from Ref. [1] summarised the workflow for computing the SHG (or higher-harmonics) in a energy range [math]\displaystyle{ [\Omega_1,\Omega_2] }[/math].
Step 1: Independent-particle approximation
Step 2: Hartree+Screened exchange approximation (BSE level)
- ↑ C. Attaccalite and M. Gruning Rev. B, 88, 235113 (2013)