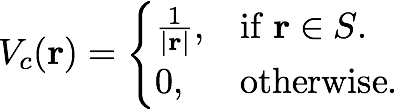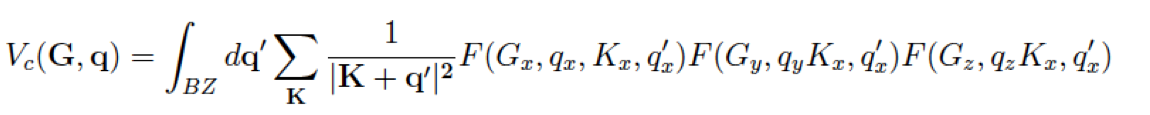How to treat low dimensional systems

In this tutorial you will learn for a low-dimensional (2D) material how to:

• Avoid numerical divergence using the Random Integration Method (RIM)
• Generate a truncated coulomb potential with a box-like cutoff to eliminate the image-image interactions
• Use the truncated coulomb potential in the GW calculation
• Use the truncated coulomb potential in the BSE calculation
• Analyze the difference with corresponding calculations without the use of a truncated potetnial

Prerequisites

• Complete the Generating the Yambo databases tutorial
• SAVE folder for 2D hBN.
• yambo executable
• ypp executable
• Run Initialization

Avoid numerical divergence using the Random Integration Method

In DFT runs of low-dimensional materials low dimensional k-grids are generally used. (i.e. NxNx1 for a 2D sheet perpendicular to the z direction) This can create numerical problems in the convergence of the many-body results due to the divergence of the coulomb potential (which appears in all the main equations, see i.e. the exchange self-energy equation) for small q.

To eliminate this problem YAMBO uses the so-called Random Integration Method which means to use a Monte Carlo Integration with Random Q-points whose number RandQpts is given in input.

Create the input to generate the ndb.RIM database

$ yambo -F yambo_RIM.in -r 

 RandQpts= 1000000            # [RIM] Number of random q-points in the BZ
 RandGvec= 1            RL    # [RIM] Coulomb interaction RS components 

Close input and Run yambo

$ yambo -F yambo_RIM.in -J 2D

At the end a new database is present in the 2D directory

 ndb.RIM

Note that RandGvec=1 means use RIM only for the G=0 but if it can be used also for higher components (convergence of the observable should be checked).

$ yambo -F yambo_cut2D.in -x -r 

Unfortunately the presence of the numerical instability is evident only using denser k-grids with respect to that one used in this Tutorial (6x6x1). To see it you should generate other SAVE directories with denser k-grids and check i.e. the HF gap. Here we report the HF gap calculated with different k-grids without (noRIM) and with Random Inetgration Method (RIM) to show the problem.

            noRIM   RIM
 6x6x1  
 12x12x1
 15x15x1
 45x45x1 

So home message : use always the RIM in MB simulations of low-dimensional materials.

Generate a truncated coulomb potential/ndb.cutoff database (yambo -r)

To simulate an isolated nano-material a convergence with cell vacuum size is in principle required, like in the DFT runs. The use of a truncated Coulomb potential allows to achieve faster convergence eliminating the interaction between the repeated images along the non-periodic direction (see i.e. D. Varsano et al Phys. Rev. B and .. ) In this tutorial we learn how to generate a box-like cutoff for a 2D system with the non-periodic direction along z.

In YAMBO you can use :

spherical   cutoff (for 0D systems)  
cylindrical cutoff (for 1D systems) 
box-like    cutoff (for 0D, 1D and 2D systems)

The Coulomb potential with a box-like cutoff is defined as

Then the FT component is

where

For a 2D-system with non period direction along z-axis we have

Important remarks:

• the Random Integration Method (RIM) is required to perform the Q-space integration
• for sufficiently large supercells a choose L_i slightly smaller than the cell size in the i-direction ensures to avoid interaction between replicas

Creation of the input file:

$ yambo -F yambo_cut2D.in  -r

Open the input file yambo_cut2D.in

Change the variables inside as:

RandQpts= 1000000          # [RIM] Number of random q-points in the BZ
RandGvec= 100        RL    # [RIM] Coulomb interaction RS components

CUTGeo= "box z"            # [CUT] Coulomb Cutoff geometry: box/cylinder/sphere X/Y/Z/XY..
% CUTBox
 0.00     | 0.00     | 32.0    |        # [CUT] [au] Box sides

Close the input file

Run yambo:

$ yambo -F  yambo_cut2D.in  -J 2D

in the directory 2D you will find the two new databases

ndb.RIM		ndb.cutoff